Deep Dive: How Math Practices 7 and 8 Power Student “Lightbulb” Moments
Our final series installment on how Math Practices 7 and 8 give students opportunities to work through mistakes and make their own pathways and breakthroughs.
Deep Dive: How Math Practices 7 and 8 Power Student “Lightbulb” Moments
Our final series installment on how Math Practices 7 and 8 give students opportunities to work through mistakes and make their own pathways and breakthroughs.
In this four-part series, the EdReports mathematics team explores the Standards for Mathematical Practice and why they're essential for every student to learn and grow. Read the rest of the series here:
- How Math Practices 1–3 Help All Students Access Math Learning and Build Skills for the Future
- Mathematics for All—How Modeling Transforms Student Learning
- How Math Practices 5 and 6 Build Student Confidence and Ownership of Their Learning
When I was in school, my math teachers would often kick off a new topic by telling us the relevant formula or shortcut upfront—then we’d get straight down to practicing the procedure. Looking back, it was kind of like a movie spoiler: we knew the ending, but we missed out on getting invested in the why and the how of the story and character development along the way.
The Math Practices set students up to work through mistakes, try a range of approaches, and find different pathways toward making their own conceptual breakthroughs.
To grow toward college- and career-ready proficiency, students need opportunities to actively engage in their own mathematical exploration and experimentation. That’s exactly what the Standards for Mathematical Practice prompt us to do as educators: set students up to work through mistakes, try a range of approaches, and find different pathways toward making their own conceptual breakthroughs.
Math Practices 7 and 8 epitomize this, empowering learners to uncover the underlying structures of problems, and to prove—and even create—methods and formulas for themselves.
Math Practice 7 (MP7): Look for and make use of structure
| Example of MP7 in a 7th Grade Classroom Students engage with the following problem. They’ve learned and practiced applying the formula C = πd (circumference = pi multiplied by diameter) in previous lessons. Find the circumference of the circle. The sides of the square are 3 inches long. |
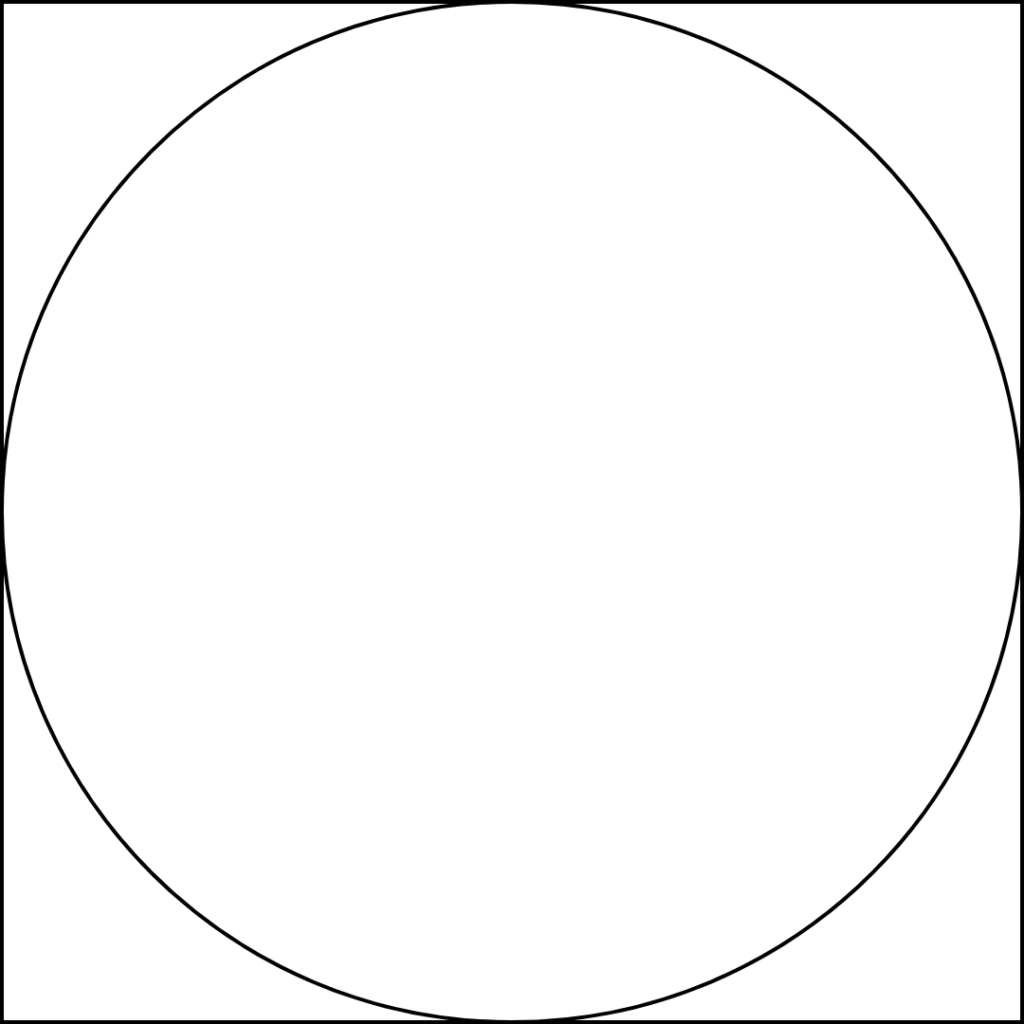
| Learners might initially see the two shapes as unrelated and not know where to start. But by using their existing knowledge, they can make use of the shapes’ structures to solve the problem. Students know that all sides of a square are equal (K–5 content standards). They can also visualize or draw the diameter of the circle (K–5 content standards) and see that it has the same value as the sides of the square. |
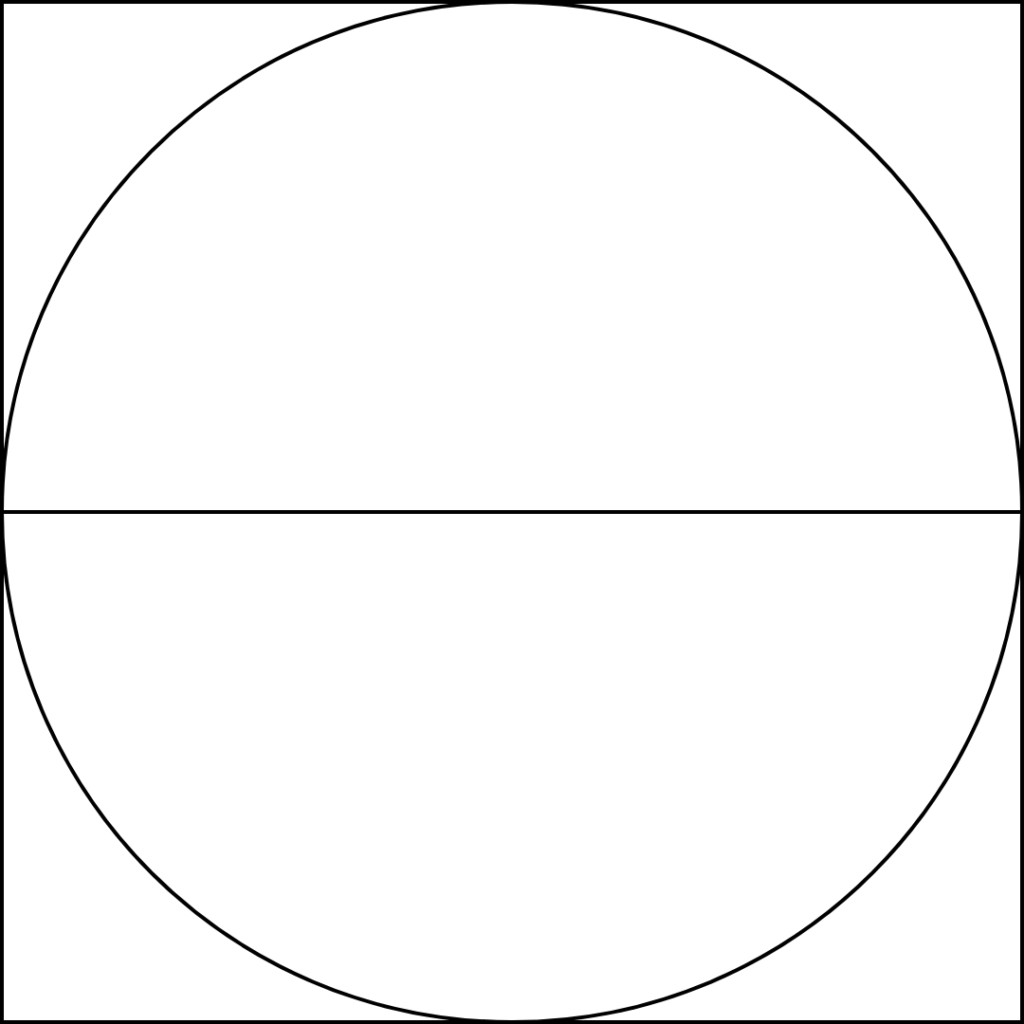
| Once students understand that the sides of the square and the diameter of the circle are equivalent, they can calculate the circumference of the circle using the formula C = πd. |
Why this Practice is important:
Students engaging in Math Practice 7 look for patterns or structures to make generalizations and solve problems, seek out multiple approaches when analyzing problems, and find ways to simplify complex expressions and representations.
MP7 is all about building the habit of looking carefully to see how the parts of a mathematical object work together. As well as solving the problem in front of them, this allows students to activate and deepen prior knowledge by connecting a problem to related mathematical objects and concepts.
Analyzing and questioning structures is an essential, real-world skill that transfers across subjects and beyond—whether it’s the structure of a cylinder, a cell, a sonnet, or a society.
What’s more, analyzing and questioning structures is an essential, real-world skill that transfers across subjects and beyond—whether it’s the structure of a cylinder, a cell, a sonnet, or a society.
Math Practice 8 (MP8): Look for and express regularity in repeated reasoning
| Example of MP8 in a 5th Grade Classroom Students solve the problems below to find the missing numbers: 1/6 x ? = 20 2/6 x ? = 20 3/6 x ? = 20 4/6 x ? = 20 5/6 x ? = 20 6/6 x ? = 20 This set of problems allows students to engage with MP8 via a “guess-check-generalize” approach. They guess a method to find the missing value in the first expression and check if the method works; if it does, they can use repeated reasoning to apply it to the other expressions to find out if it generalizes. Math Practice 8 calls for students to “maintain oversight of the process, while attending to the details." In this case, oversight means noticing how the answer changes as the fractions increase across problems. At the same time, students attend to the detail of whether their method produces an accurate answer in each instance. |
Why this Practice is important:
Math Practice 8 is about investigating general approaches to a type or category of problem rather than solving a single, standalone problem. So, students use repeated calculation and reasoning in order to understand or create a method, formula, shortcut, or algorithm that can be applied to a given group of related problems.
For example, elementary students might add 2 + 2, then 2 + 2 + 2, and so on, to understand that multiplication is a shortcut for repeated addition. Older learners might substitute a range of different inputs into a formula to test whether it holds true across all contexts.
When learners prove or discover a method for themselves through repeated reasoning, they’re engaging deeply with mathematical concepts and procedures.
MP8 builds student confidence and belief, both in math and in their own proficiency. When learners prove for themselves that a method works—or even discover their own method through repeated reasoning—they’re engaging deeply with mathematical concepts and procedures.
That kind of engagement can’t be accessed by introducing a rule or method and telling students that it “just works.” They know and believe that it works because they tried it out themselves.
The Role of Instructional Materials and How EdReports Reviews for the Math Practices
EdReports’ educator reviewers consider a range of factors when evaluating materials for Math Practices 7 and 8 in a K-12 curriculum review. This includes looking for problems that are open and challenging enough to require students to think about different approaches and checking whether a program provides a variety of examples that explicitly focus on patterns and repeated reasoning.
Math Practices 7 and 8 encourage productive struggle in order for students to earn the thrill and reward of their own “lightbulb” moments.
Both Practices encourage productive struggle in order for students to earn the thrill and reward of their own “lightbulb” moments. To engage learners in Math Practice 7, materials should avoid telegraphing explicitly that something could or should be done to the structure of a problem; students should have multiple chances to make those structural “aha” breakthroughs themselves.
When considering Math Practice 8 in instructional materials review, one pitfall is for programs to offer hints or procedures that take students straight to a shortcut or rule of thumb. Again, we want materials to give students extensive opportunities to discover methods independently through repeated reasoning.
The Impact of Math Practices 7 and 8
Examining and interrogating structures; experimenting with multiple strategies to solve a problem; testing and iterating on your own approaches and hypotheses. These are the kinds of invaluable, real-world skills students need to learn and grow into proficient mathematicians prepared for college and careers.
Instead of giving spoilers, Math Practices 7 and 8 put students front and center in finding their own paths and shaping their own stories.
Related Resources
Deep Dive: How Math Practices 5 and 6 Build Student Confidence and Ownership of Their Learning
The next installment in our series explores how Math Practices 5 and 6 help students approach problems with confidence and express their conceptual understanding with precision.
Deep Dive: How Math Practices 1–3 Help All Students Access Math Learning and Build Skills for the Future
Part one of our series on the Mathematical Practices focuses on why they matter for student learning, and the role high-quality instructional materials play in supporting teachers to incorporate them meaningfully into the classroom.
Deep Dive: Mathematics for All – How Modeling Transforms Student Learning
Educator Tim Truitt shares why Math Practice 4 is integral to making mathematics relevant and meaningful for each and every student.




